Exact solutions for social and biological contagion models on mixed directed and undirected, degree-correlated random networks

More recent
Temporal patterns of happiness and information in a global social network: Hedonometrics and Twitter

More or equal citational love
Ratioing the President: An exploration of public engagement with Obama and Trump on Twitter

Less recent
Direct, physically motivated derivation of the contagion condition for spreading processes on generalized random networks

Less or equal citational love
Interpretable bias mitigation for textual data: Reducing gender bias in patient notes while maintaining classification performance

Exact solutions for social and biological contagion models on mixed directed and undirected, degree-correlated random networks

J. L. Payne, K. D. Harris, and P. S. Dodds

Physical Review E, 84, 016110, 2011

arXiv version | arXiv page | journal version | journal page

Times cited: 24

Abstract:

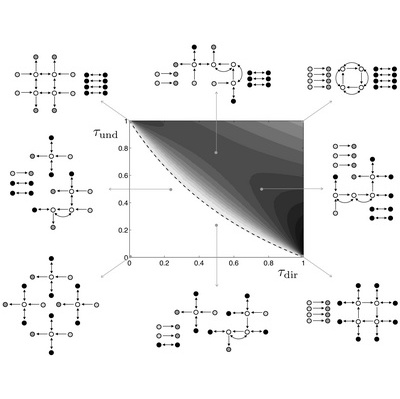
We derive analytic expressions for the probability and expected size of global spreading events starting from a single infected seed for a broad collection of contagion processes acting on random networks with both directed and undirected edges and arbitrary degree-degree correlations. Our work extends previous theoretical developments for the undirected case, and we provide numerical support for our findings by investigating an example class of networks for which we are able to obtain closed-form expressions.

This is the default HTML.
You can replace it with your own.
Include your own code without the HTML, Head, or Body tags.

BibTeX:

@Article{payne2011a,
  author = 	 {Payne, Joshua L. and Harris, Kameron D. and Dodds, Peter Sheridan},
  title = 	 {Exact solutions for social and biological contagion models on mixed directed and undirected, degree-correlated random networks},
  journal =      {Phys. Rev. E},
  key = 	 {contagion},
  year = 	 {2011},
  volume =       {84},
  pages  =       {016110},
}