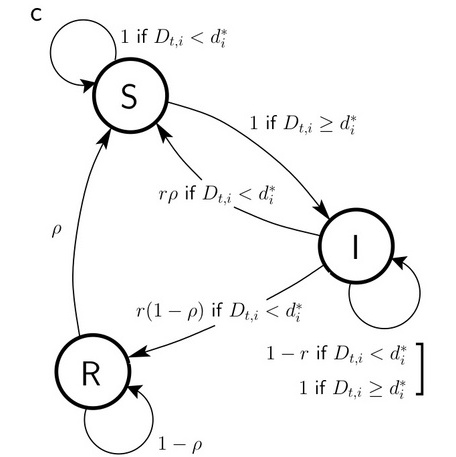
We present a model of contagion that unifies and generalizes threshold models of social contagion and epidemiological models of disease spreading. Our model incorporates individual memory of exposure to a contagious entity (e.g., a rumor or disease), variable magnitudes of exposure (dose sizes), and heterogeneity in the susceptibility of individuals. Through analysis and simulation, we examine in detail the case where individuals may recover from an infection and then immediately become susceptible again (analogous to the so-called SIS model). We identify three basic classes of contagion models which we call epidemic threshold, vanishing critical mass, and critical mass classes respectively, where each class of models corresponds to different strategies for prevention or facilitation. We find that the conditions for a particular contagion model to belong to one of the these three classes depend only on memory length and the probabilities of being infected by one and two exposures respectively. These parameters are in principle measurable for real contagious influences or entities, thus yielding empirical implications for our model. We also study the case where individuals attain permanent immunity once recovered, finding that epidemics inevitably die out but may be surprisingly persistent when individuals possess memory.