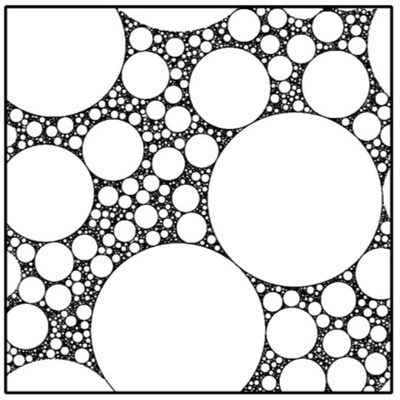
We consider growing spheres seeded by random injection in time and space. Growth stops when two spheres meet leading eventually to a jammed state. We study the statistics of growth limited by packing theoretically in $d$ dimensions and via simulation in $d$=2, 3, and 4. We show how a broad class of such models exhibit distributions of sphere radii with a universal exponent. We construct a scaling theory which relates the fractal structure of these models to the decay of their pore space, a theory which we confirm via numerical simulations. The scaling theory also predicts an upper bound for the universal exponent and is in exact agreement with numerical results for $d$=4.